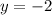Question

Given the graph of trapezoid QUAD and its reflection.

Use the coordinates of the images' vertices, U and U' to algebraically find the line of reflection. In your final answer, include all of your work.

Answer 1

Answer:
`y=-2`

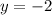
Explanation:

A reflection is a kind of rigid transformation where each point in a shape moves at an equal distance on the opposite side across the given line of reflection. It basically produces a mirror image of the original figure.

From the given picture, it can be seen that the coordinates of U and U'(2,0) are (1,2) and (1,-6) respectively.

Since x coordinate is same for both the coordinates.

let the coordinate for the mid point is (1,a).

Then ,
`a=(-6+2)/(2)=(-4)/(2)=-2`

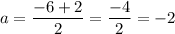
Thus, coordinate of mid point = (1,-2)

Since each point in a shape moves at an equal distance on the opposite side across the given line of reflection, therefore we have the line of reflection =
`y=-2`

Answer 2

Y = -2

Explanation:

A reflection will produce a mirror image of the shape. Both shapes will be equal distance from the line of reflection and perpendicular to it.

To find it, connect Q and Q' with a line segment. Do the same to all corresponding vertices. The line of reflection will be perpendicular to these segments. This means it is a horizontal line through the y-axis and of the form y=b.

To find the y-coordinate b, subtract the y-values of U and U' then divide by 2.

U is (1,2) and U' is (1,-6) so (2--6)/2 = 8/2=4. The line of reflection is 4 units below U and 4 units above U'. This is -2. The line of reflection is y = -2.