Question

Given: △ABC, E∈ AB m∠ABC=m∠ACE AB=34, AC=20 Find: AE

Answer 1

AE = 11.76 units

Explanation:

For better understanding of the solution, see the attached figure :

Given : E ∈ AB, m∠ABC = m∠ACE, AB = 34 and AC = 20

To find AE :

In ΔABC and ΔACE,

m∠ABC = m∠ACE ( Given )

∠A = ∠A ( Common angle for both the triangles )

By AA postulate of similarity of triangles, ΔABC ~ ΔACE

So, proportion of the corresponding will be equal.

`\implies (AC)/(AB)=(CE)/(BC)=(AE)/(AC)\\\\\implies(AC)/(AB)=(AE)/(AC)\\\\\implies(20)/(34)=(AE)/(20)\\\\\implies AE=(20* 20)/(34)\approx 11.76`

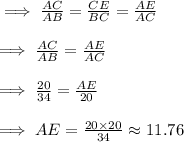
Hence, AE = 11.76 units