Question

Jin needs to fence in his rectangular backyard. The fence will have one long section away from, but parallel to, the length of his house and two shorter sides connecting that section to the house. The length of Jin’s house is (3x+ 4) yd and the area of his backyard is (9x2+ 15x+ 4) yd^2. How many yards of fencing will Jin need?

A. (6x+ 2) yd C. (9x+ 6) yd
B. (9x+ 9) yd D. (12x+ 10) yd hint: ^2 means squared.

Answer 1

Answer: C. 9x+6 yd

Explanation:

Given: The length of Jin's house=
`3x+4\ yd`

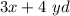
The area of Jin's backyard=
`9x^2+15x+4\ yd^2`

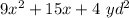
By middle splitting method, The area of Jin's backyard=
`9x^2+12x+3x+4\ yd^2=(3x+1)(3x+4)\ yds`

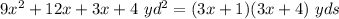
To find the width of the backyard, divide the area by the length, we get

Width
`=((3x+1)(3x+4))/((3x+4))=3x+1`

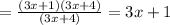
According to the question, only three sides need to fence

`Fencing=3x+1+3x+1+3x+4=9x+6\ yd`