Answer:
vertex is (9,-21)
Explanation:
we are given function as
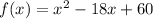
we can use vertex form of parabola
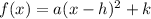
So, firstly we will complete x square
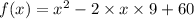
we can sue formula of square
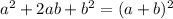
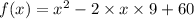
so, we will add and subtract 9^2
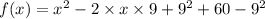
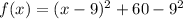
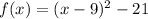
now, we can compare it with vertex form of formula
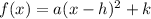
so, we get
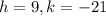
So, vertex is (9,-21)