Answer:
$1573.31
Explanation:
We have been given that an electronics store to be paid back with monthly at a 14.4 APR compounded monthly.
As Rufus will begin to make payments after 4 months, so we will find the total amount after 4 months using compound interest formula.
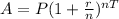 , where,
, where,
A = Final amount after T years,
P = Principal amount,
r = Interest rate in decimal form,
n = Number of times interest in compounded per year,
T = Time in years.
Let us convert given interest rate in decimal form.
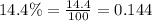
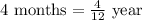
Upon substituting our given values in compound interest formula we will get,
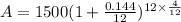
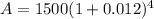
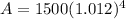
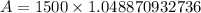
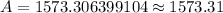
Therefore, Rufus will owe $1573.31 when he begins making payment.