Question

If M is the midpoint of XY, find the coordinates of Y if X(-1,-3) and M(0.5,-1.6)

Answer 1

The coordinates of Y are (2,-0.2)

Explanation:

Let the coordinates of Y be (x,y)

Since M is the midpoint of XY, so

`M_(x) =(X_(x)+Y_(x))/(2)`

`0.5 =(-1+Y_(x))/(2)`

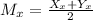
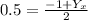
Multiply both sides by 2

`0.5*2 =(-1+Y_(x))/(2)*2`

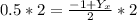
Cancel out the 2's from the top and bottom on the right side

`1 =-1+Y_(x)`

Add 1 to both sides

`1+1 =-1+Y_(x)+1`

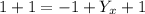
Cancel out -1 and +1 on the right side

`2 =Y_(x)`

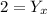
Flip the sides

`Y_(x)=2`

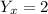
Similarly,

`M_(y) =(X_(y)+Y_(y))/(2)`

`-1.6 =(-3+Y_(y))/(2)`

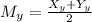
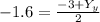
Multiply both sides by 2

`-1.6*2 =(-3+Y_(y))/(2)*2`

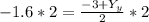
Cancel out the 2's on the top and bottom of the right side

`-3.2 =-3+Y_(y)`

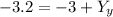
Add 3 to both sides

`-3.2+3 =-3+Y_(y)+3`

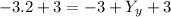
Cancel out -3 and +3 on the right side

`-0.2 =Y_(y)`

Flip the sides

`Y_(y)=-0.2`

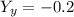
So, the coordinates of Y are (2,-0.2)