Question

( 3 x 2 ) ^ 4 x 3 ^ -3 / 2 ^ 3 x 3 =

Simplify the exponential expression

Answer 1

2

Explanation:

`((3*2)^4*3^(-3))/(2^3*3)`

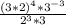
we start simplifying by removing the parenthesis

Multiply the exponents inside the the parenthesis

3^4 * 2^4

`(3^4*2^4*3^(-3))/(2^3*3)`

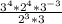
Now we apply exponential property

a^m * a^n = a^ (m+n)

3^4 * 3^-3 = 3^ (4-3) = 3^1

3 or 3^1 are same

`(3^1*2^4)/(2^3*3^1)`

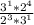
3^1 at the top and bottom so we cancel it out

\frac{2^4}{2^3}

we apply log property . a^m / a^n = a^m-n

Now subtract the exponents

2^(4-3) = 2^1 = 2