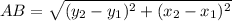Question

Suppose two points on a grid are not on the same horizontal or vertical line. Describe how you can use the Pythagorean theorem to find the distance between the points without measuring

Answer 1

`AB=\sqrt{(y_(2)-y_(1))^(2)+(x_(2)-x_(1))^(2)}`

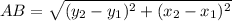
Explanation:

Given two points in grid which are not on the same horizontal line or vertical line. Hence, it will be like as shown in figure.

Let
`A(x_(1),y_(1)) and B(x_(2),y_(2))` are the points on grid.

AO=(y-coordinate of A)-(y-coordinate o B)

=
`y_(2)-y_(1)`

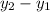
OB=(x-coordinate of A)-(x-coordinate o B)

=
`x_(2)-x_(1)`

Hence, By Pythagoras theorem, distance between the points A and B i. AB can be calculated as

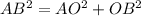
`AB^(2)=AO^(2)+OB^(2)`

`AB^(2)=(y_(2)-y_(1))^(2)+(x_(2)-x_(1))^(2)`

⇒
`AB=\sqrt{(y_(2)-y_(1))^(2)+(x_(2)-x_(1))^(2)}`