What is the perimeter of a parallelogram, if its area is 24 cm2 and and the distances between the point of intersection of the diagonals and the sides are 2 cm and 3 cm?

Question

asked Sep 27, 2020 97.9k views

1 Answer

Willome · Answer 1 · 2020-10-01T02:00:06+0000

Answer:

p=20cm

Explanation:

Area of parallelogram is :
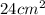
24cm^(2)

Since, it is a parallelogram, the four triangles are created by drawing diagonals of equal area=
(24)/(4)=6cm^(2) .

Now, Height of the one triangle is 2cm and the other is 3 cm.

Therefore, From ΔODC,

Area=
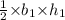
$(1)/(2){*}b_(1){*}h_(1)$

=
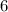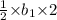
$(1)/(2){*}b_(1){*}2$

b_(1)=6

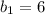
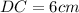
DC=6cm

Again, from ΔOAD,

Area=
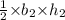
$(1)/(2){*}b_(2){*}h_(2)$

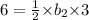
$6=(1)/(2){*}b_(2){*}3$

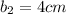
b_(2)=4cm

AD=4cm

Now, opposite sides of parallelogram are equal, therefore,AD=BC=4cm and DC=AB=6cm.

Perimeter of parallelogram= Sum of all the sides

=AB+BC+CD+AD

=6+4+6+4

=20cm