Question

Can you help with this one too.

Answer 1

Odd, Even

Explanation:

A function is said to be an Even function if it follows f(-x) = f(x).

A function is said to be an Odd function if it follows f(-x) = -f(x).

It means we can plug -x in place of x and check if it comes out to be f(x) or -f(x) i.e. y or -y?

Given y = sin³x/cos³x and y = 1/sec³x

`y = sin^3x/cos^3x\\f(x) = sin^3x/cos^3x\\f(-x) = sin^3(-x)/cos^3(-x)\\f(-x)= (sin(-x))^3/(cos(-x))^3\\f(-x)=(-sin(x))^3/(cos(x))^3\\f(-x)=-sin^3x/cos^3x\\f(-x)=-f(x)\\Odd\; function`

`y=1/sec^3x\\f(x)= 1/sec^3x\\f(x)=cos^3x\\f(-x)=cos^3(-x)\\f(-x)=(cos(-x))^3\\f(-x)=(cos\;x)^3\\f(-x)=cos^3x\\f(-x)=f(x)\\Even\; function`

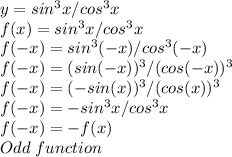
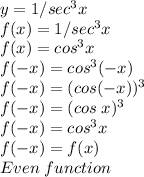
Hence, first function is Odd, second function is Even.