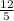Question

The coordinates of point F are (8, 4) and the coordinates of point G are (−4, 9) .

What is the slope of the line that is perpendicular to FG⎯⎯⎯⎯⎯?



Enter your answer as a simplified fraction in the box.

Answer 1

The slope of the line that is perpendicular to FG is
`(12)/(5)`

Explanation:

step 1

Find the slope of line FG

we have

`F(8,4),G(-4,9)`

`m=(9-4)/(-4-8)`

`m=(5)/(-12)`

`m=-(5)/(12)`

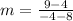
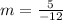
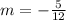
step 2

Find the slope of the line that is perpendicular to FG

we know that

If two lines are perpendicular, then the product of their slopes is equal to -1 ( the slopes are inverse reciprocal each other)

so

`m1*m2=-1`

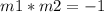
we have

`m1=-(5)/(12)`

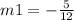
substitute and solve for m2

`(-(5)/(12))*m2=-1`

`m2=(12)/(5)`

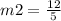
therefore

The slope of the line that is perpendicular to FG is
`(12)/(5)`