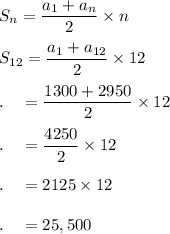Answer: $25,500
Explanation:
The given sequence {1300, 1300+150, ... } provides the following information:
- the first term (a₁) = 1300
- the difference (d) = 150
We can use the information above to find the explicit rule of the sequence:
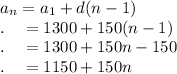
We can use the explicit rule to find the 12th term (a₁₂)
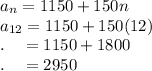
Next, we can input the first and last term of the sequence into the Sum formula: