Answer:
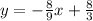
Or

Explanation:
The line passes through
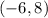
The x-intercept being 3 means the line also passes through
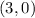 .
.
We can use the formula,

The slope of the given line is
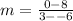
This simplifies to
 .
.
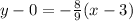
This gives
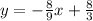
This is the slope-intercept form.
When we multiply through by 9, we get,
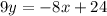
Or
