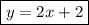The slope-intercept form:

m - slope
b - y-intercept
The formula of a slope:
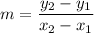
We have the points (2, 6) and (-4, -6). Substitute:
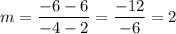
Therefore we have the equation
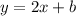
Put the coordinates of the point (2, 6) to the equation:
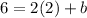
 subtract 4 from both sides
subtract 4 from both sides
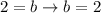
Answer: