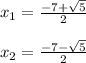Question

What are the solutions of the equation (2x + 3)2 + 8(2x + 3) + 11 = 0? Use u substitution and the quadratic formula to solve

Answer 1

The given expression in single variable is:

`(2 x +3)^2 +8 * (2 x +3) +11=0\\\\ 4x^2 +9 +2 * 2 x  * 3+8 * 2 x+8 * 3+11=0\\\\4 x^2+12 x+16 x+9+24+11=0\\\\4x^2+28 x+44=0\\\\4 * (x^2+7 x+11)=0\\\\x^2+7 x+11=0\\\\ x=(-7\pm √(7^2-4 * 11 * 1))/(2 * 1)\\\\x=(-7\pm √(49-44))/(2)\\\\x=(-7\pm √(5))/(2)`

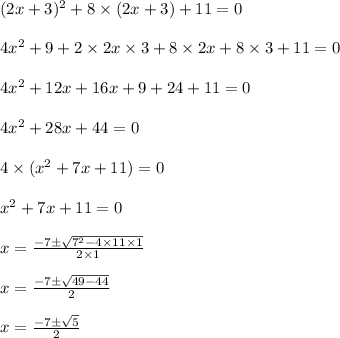
⇒Used Distributive property of Multiplication with respect to Addition:

a × (b+c)=a ×b+a × c

⇒For, a Quadratic expression of the form

Ax² +Bx +C=0

Discriminant=D=B²-4 AC

`x=(-B\pm √(D))/(2 A)`

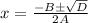
There are two zeroes which are

`x_(1)=(-7+ √(5))/(2)\\\\x_(2)=(-7- √(5))/(2)`