Question

What are the approximate values of the minimum and maximum points of f(x) = x5 − 10x3 + 9x on [-3,3]?

Answer 1

answer is A for edmentum ppl ;)

Step-by-step explanation:

Answer 2

Minimum : -37 at x=2.4 and

Maximum = 37 at x=-2.4.

Step-by-step explanation:

Given:

`f(x)=x^5-10x^3+9x`; [-3,3]

Step-by-step explanation:

In order to find minimum/maximum of a function, we need to find the first derivative of the function and then set it equal to 0 to get critical points.

Therefore,

`f'(x)=5x^4-30x^2+9`

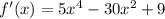
Setting derivative equal to 0, we get

`5x^4-30x^2+9=0`

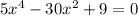
On applying quadratic formula, we get

x=2.4, -2.4, -0.7, 0.7.

So, those are critical points of the given function.

Plugging the values x=2.4, -2.4, -0.7, 0.7, -3 and 3 in above function, we get

f(2.4)=(2.4)^5-10(2.4)^3+9(2.4)= -37.01376 : Minimum.

f(-2.4)=(-2.4)^5-10(-2.4)^3+9(-2.4)= 37.01376 : Maximum.

f(0.7)=(0.7)^5-10(0.7)^3+9(0.7) = 3.03807

f(-0.7)=(-0.7)^5-10(-0.7)^3+9(-0.7) = -3.03807

f(-3)=(-3)^5-10(-3)^3+9(-3) =0

f(3)=(3)^5-10(3)^3+9(3) =0

Therefore the approximate values of the minimum and maximum points of f(x) =
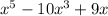`x^5- 10x^3+ 9x` on [-3,3] are:

Minimum : -37 at x=2.4 and

Maximum = 37 at x=-2.4.