Answer:
(a) we are given two functions f(x) and g(x) as:
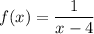 and
and
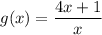
Now we find out the composition maps fog and gof as:
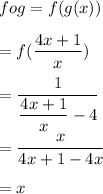
Hence,

similarly we find gof.
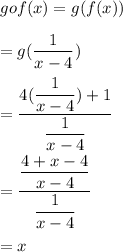
hence,
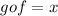
as fog=gof=x this means that f and g are inverse of each other.
(b) the domain of f is given by: (-∞,4)∪(4,∞)
since, f is defined all over the real line except at 4 ; at 4 the function is not defined as the denominator is zero.
also g is defined everywhere except at 0; since at 0 denominator is 0.
hence, domain of g(x) is (-∞,0)∪(0,∞).
domain of the composition fog is the domain of the function g(x) ( as for defining fog we need to evaluate function g(x) first and then f(g(x)) ).
and domain of function gof is equal to domain of function f(x) ( as for defining gof we need to evaluate function f(x) first and then g(f(x)) ).
hence domain of fog=(-∞,0)∪(0,∞).
and domain of gof=(-∞,4)∪(4,∞)