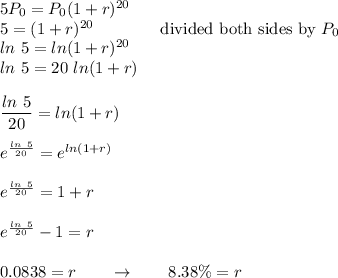Question

A sum of money becomes 5 times itself in 20 years at a certain rate of compound interest. What rate of interest per annum becomes four times itself?

Answer 1

Answer: 8.38%

Explanation:

The formula for interest compounded annually is:
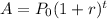`A = P_0(1 + r)^t` where

• A is the amount accrued (ending balance)
• P₀ is the initial amount invested
• r is the interest rate
• t is the time (in years)

With the given information, we have:

• A = 5P₀
• P₀ = P₀
• r = ?
• t = 20

`5P_0=P_0(1+r)^(20)\\5 = (1+r)^(20)\qquad \qquad \text{divided both sides by}\ P_0\\ln\ 5=ln(1+r)^(20)\\ln\ 5=20\ ln(1+r)\\\\(ln\ 5)/(20)=ln(1+r)\\\\e^{(ln\ 5)/(20)}=e^(ln(1+r))\\\\e^{(ln\ 5)/(20)}=1 + r\\\\e^{(ln\ 5)/(20)}-1 = r\\\\0.0838 = r\qquad \rightarrow \qquad 8.38\% = r`