Answer: The linear expression is x+4.
Step-by-step explanation: We are given to create a linear expression and also to create equivalent expressions and explain your other expressions are equivalent to the original one.
Let the linear expression be
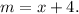
And also, equivalent expressions be
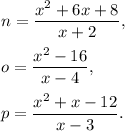
Since,

Thus, these three expressions 'n', 'o' and 'p' are equivalent to the original expression 'm'.