Question

Consider a box that contains 14 red balls,12 blue balls,and 9 yellow balls.A ball is drawn at random and the color is noted and then put back inside the box.Then, another ball is drawn at random.Find the probability that: a.Both are blue b.The first is red and the second is yellow

Answer 1

a.
`\text{Probability of getting two blue balls}=(144)/(1225)=0.117551`

b.
`P(\text{Red then yellow})=(18)/(175)=0.1028571`

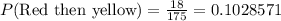
Explanation:

We have been given that a box contains 14 red balls,12 blue balls,and 9 yellow balls.A ball is drawn at random and the color is noted and then put back inside the box.Then, another ball is drawn at random.

a. Since the balls are being replaced after each draw, so the probability of any two events will be independent.

`\text{Probability}=\frac{\text{The number of favorable outcomes}}{\text{Total number of possible outcome}}`

`\text{Probability of getting a blue ball}=(12)/(14+9+12)`

`\text{Probability of getting a blue ball}=(12)/(35)`

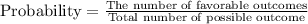
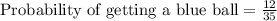
As ball is replaced so number of total balls and each color ball will be same. The probability of getting second blue ball will be 12/35. So by the multiplication rule of probability for independent events:

`\text{Probability of getting two blue balls}=(12)/(35)*(12)/(35)`

`\text{Probability of getting two blue balls}=(144)/(1225)`

`\text{Probability of getting two blue balls}=0.117551`

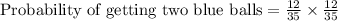
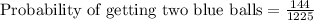
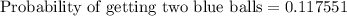
Therefore, probability of getting two blue balls will be 0.117551.

b. Let us find probability of getting a red ball.

`\text{Probability of getting a red ball}=(14)/(14+9+12)`

`\text{Probability of getting a red ball}=(14)/(35)`

`\text{Probability of getting a yellow ball}=(9)/(14+9+12)`

`\text{Probability of getting a yellow ball}=(9)/(35)`

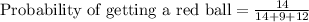
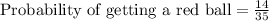
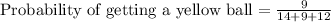
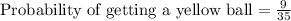
By the multiplication rule of probability for independent events probability of getting a red then yellow ball will be:

`P(\text{Red then yellow})=(14)/(35)*(9)/(35)`

`P(\text{Red then yellow})=(2)/(5)*(9)/(35)`

`P(\text{Red then yellow})=(18)/(175)`

`P(\text{Red then yellow})=0.1028571`

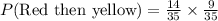
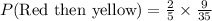
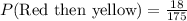
Therefore, probability of getting first a red and then a yellow ball is 0.1028571.

Answer 2

a. The probability of both balls are blue is
`(144)/(1225)`.

b. The probability of getting first red and second yellow ball is
`(18)/(175)`.

Explanation:

It is given that the box contains 14 red balls,12 blue balls,and 9 yellow balls. The total number of balls is

`14+12+9=35`

The probability is defined as

`p=\frac{\text{Favorable outcomes}}{\text{Total number of outcomes}}`

Probability of getting red ball =
`(14)/(35)`

Probability of getting blue ball =
`(12)/(35)`

Probability of getting yellow ball =
`(9)/(35)`

It is given that a ball is drawn at random and the color is noted and then put back inside the box. It means first event will not effect the probability of second event.

a.

The probability of both balls are blue is

`(12)/(35)* (12)/(35)=(144)/(1225)`

b.

The probability of getting first red and second yellow ball is

`(14)/(35)* (9)/(35)=(18)/(175)`