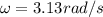Part A)
If water will not spill from the bucket then at minimum speed condition the value of normal force on water will be zero at the top position
so now we will have
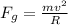
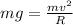
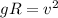

now we know that
R = 1 m
g = 9.8 m/s^2
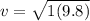
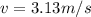
PART B)
for minimum angular speed we know the relation between angular speed and linear speed as
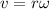
now we know that
r = 1 m
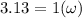
so angular speed will be