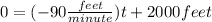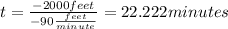Question

A hot air balloon descends from an altitude of 2,000 feet at a constant rate of 90 feet per minute. The graph shows the altitude of the balloon over time. Write a linear function in the form y = mx + b to represent the situation.

Answer 1

y=90x+2,000

Explanation:

90 is the rate of change because the hot air balloon descends 90 feet per minute.

And 2,000 is the starting point because the hot air balloon started the descent at 2,000 feet.

Answer 2

`y(t)=(-90(feet)/(minute))t+2000feet`

Explanation:

Given the linear function in the form

`y=mx+b`

We are going to represent the situation using the linear function.

We are going to call the variable ''x'' time. We can write x = t ⇒

`y=mt+b`

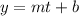
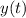`y(t)` will be the altitude function that depends on the variable ''t'' that is time in minutes.

In the instant
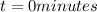`t=0minutes` the hot air balloon has an altitude of 2000 feet ⇒

`y(0)=2000feet=m(0)+b=b` ⇒
`b=2000feet`

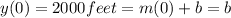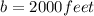
We can think that the slope ''m'' is the constant rate of the function.

Given that the hot air balloon descends,
`m<0` ⇒
`m=-90(feet)/(minute)`

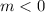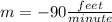
Now we write the function :

`y=mt+b\\`

`y(t)=(-90(feet)/(minute))t+2000feet`

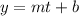
For example, when t = 0 ⇒

`y(0)=(-90(feet)/(minute)).0+2000feet=2000feet`

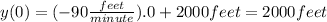
Or if we want to find the time when the hot air balloon finally descends :

`0=(-90(feet)/(minute))t+2000feet`

`t=(-2000feet)/(-90(feet)/(minute))=22.222minutes`