Question

1. The edge lengths of a right rectangular prism are

1
2
meter,
1
2
meter, and
3
4
meter. How many unit cubes with edge lengths of
1
12
meter can fit inside?

2. The base of a rectangular prism has an area of
1
8
ft2, and the height of the prism is
2
3
ft. What is the volume of the prism?

3. The edge lengths of a right rectangular prism are
2
3
meter,
1
4
meter, and
3
4
meter. How many unit cubes with edge lengths of
1
10
meter can fit inside?

Answer 1

1. 324 cubes.

2.
`(1)/(12)\text{ ft}^3`.

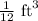3. 125 cubes.

Explanation:

Let n be the number of cubes with edge length 1/12 meter.

We have been given the lengths of edges of a right rectangular prism as 1/2 meter, 1,2 meter and 3/4 meter.

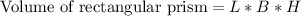`\text{Volume of rectangular prism}=L*B*H`, where,

L = Length of prism,

B = Breadth of prism,

H = Height of prism.

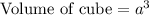`\text{Volume of cube}=a^3`, where a= Length of each edge of the cube.

The volume of n cubes with each edge 1/12 will be equal to the volume of rectangular prism.

`\text{Volume of n cubes}=\text{Volume of rectangular prism}`

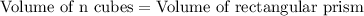
Upon substituting our given values we will get,

`n*((1)/(12))^3=(1)/(2)*(1)/(2)*(3)/(4)`

`n*(1^3)/(12^3)=(1*1*3)/(2*2*4)`

`n*(1)/(1728)=(3)/(16)`

`1728*(n)/(1728)=(3)/(16)* 1728`

`n=3* 108`

`n=324`

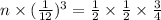
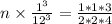
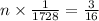
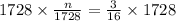
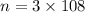
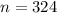
Therefore, 324 unit cubes can fit inside the given right rectangular prism.

2. Since we know that we can find volume of rectangular prism by multiplying its base area with the height of prism.

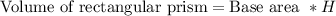`\text{Volume of rectangular prism}=\text{Base area }*H`, where,

H = Height of prism.

Upon substituting our given values we will get,

`\text{Volume of rectangular prism}=(1)/(8)(\text{ ft}^2)* (2)/(3)\text{ ft}`

`\text{Volume of rectangular prism}=(1*2)/(8*3)(\text{ ft}^2*\text{ ft})`

`\text{Volume of rectangular prism}=(2)/(24)\text{ ft}^3`

`\text{Volume of rectangular prism}=(1)/(12)\text{ ft}^3`

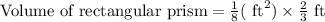
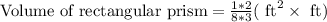
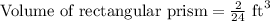
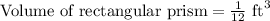
Therefore, the volume of rectangular prism will be
`(1)/(12)\text{ ft}^3`.

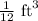3. We will solve this problem in the same way we did our 1st problem.

`\text{Volume of n cubes}=\text{Volume of rectangular prism}`

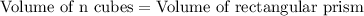
Upon substituting our given values we will get,

`n*((1)/(10))^3=(2)/(3)*(1)/(4)*(3)/(4)`

`n*(1^3)/(10^3)=(2*1*3)/(3*4*4)`

`n*(1)/(1000)=(1)/(8)`

`1000*(n)/(1000)=1000* (1)/(8)`

`n=125`

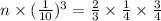
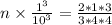
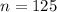
Therefore, 125 unit cubes can fit inside the given right rectangular prism.