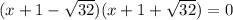Question

What equation results from completing the square and then factoring? X^+22x=31

Answer 1

21

Explanation:

Answer 2

(a)

`(x+1)^2=32`

(b)

`(x+1-√(32))(x+1+√(32)) =0`

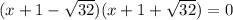
Explanation:

We are given

`x^2+2x=31`

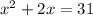
Since, we have to complete square

so, we can use formula

`a^2+2ab+b^2=(a+b)^2`

`x^2+2* x* 1=31`

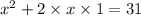
so, we can add both sides by 1^2

`x^2+2* x* 1+1^2=31+1^2`

now, we can use formula

and we get

`(x+1)^2=32`

so, equation after completing square is

`(x+1)^2=32`

now, we can solve for x

we can move all terms on left side

`(x+1)^2-32=0`

`(x+1)^2-(√(32))^2 =0`

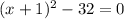
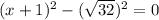
now, we can factor it

`(x+1-√(32))(x+1+√(32)) =0`