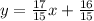Question

Complete the point-slope equation of the line through-8,-8) and (7, 9)​

Answer 1

`y = (17)/(15)x + (16)/(15)`

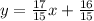
Explanation:

So the slope-intercept form is given as: y=mx+b where m is the slope and b is the y-intercept (since if you plug in 0 as x, mx will be 0 leaving only b). You can calculate the slope with the slope formula:
`slope=(y_2-y_1)/(x_2-x_1)` which is essentially
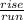`(rise)/(run)`. So for this example (-8, -8) will be (
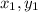`x_1, y_1`) and (7, 9) will be (
`x_2, y_2`).

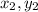Plug values into equation:

`(9 - (-8))/(7 - (-8))`

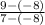
cancel out negatives

`(9+8)/(7+8)\\`

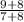
Simplify

`(17)/(15)`

The fraction is in the most simplified form unless you want to have a mixed fraction, otherwise you can't do anything else to the fraction.

so now we have the slope we have one part of the equation:
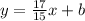`y=(17)/(15)x + b`, but now we need to solve for b. This can be done by using either of the points and plugging in x and y, but for this example I'll use (7, 9).

Plug in (7, 9) as (x, y)

`9 = (17)/(15)(7) + b`

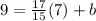
Multiply

`9 = (119)/(15)+b`

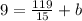
Subtract

`9-(119)/(15)=b`

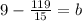
Rewrite 9 as a fraction

`(135)/(15) - (119)/(15) = b`

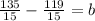
Subtract numerators

`(16)/(15) = b`

now we have both pieces of information

`y = (17)/(15)x + (16)/(15)`