Question

Please solve this thank you!

Answer 1

1024x^40

Explanation:

Apply exponent rule: (a/b)^c = a^c / b^c = (5x^9 + 3x^9)^5 / (2x)^5

Simplify:

(5x^9 + 3x^9)^5: 32768x^45

= 32768x^45 / (2x)^5

Simplify:

(2x)^5: 32x^5

= 32768x^45 / 32x^5

Divide the numbers: 32768 / 32 = 1024

= 1024x^45 / x^5

Apply exponent rule: x^a / x^b = x^a - b

x^45 / x^5 = x^45 - 5

= 1024x^45 - 5

Subtract the numbers: 45 - 5 = 40

= 1024x^40

Answer 2

`1024x^(40)`

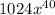
Explanation:

So the first step is to add like terms since you can simplify the numerator by adding the two values sine they have the same variable and degree.

Add like terms:

`[(8x^9)/(2x)]^5`

Divide by 2x (divide coefficient by 2, subtract coefficient degrees)

`[4x^8]^5`

Multiply exponents and raise 4 to the power of 5

`1024x^(40)`

The reason you multiply exponents is because you can think about it like this:

(4 * x * x * x * x * x * x * x * x) (this has one 4 and 8 x's because x is raised to the power of 8. Now if you do that 5 times which is what the exponent is doing you're going to have 40 x's and 8 4's. So it's essentially

(4 * x * x * x * x * x * x * x * x) * (4 * x * x * x * x * x * x * x * x) * (4 * x * x * x * x * x * x * x * x) * (4 * x * x * x * x * x * x * x * x) * (4 * x * x * x * x * x * x * x * x). If you group like terms you'll get (4 * 4 * 4 * 4 * 4) * (x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x * x). Which simplifies to 4^5 * x ^ (8 * 5) which further simplifies to the answer 1024x^40