Answer:
The money after 3 years is $5819.8735
Explanation:
We are given
monthly payment =$75
so, P=75
annuity that earns 48% APR
so, r=48%
Since, it is compounded monthly
so,
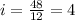 %
%
i=0.04
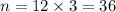
now, we can use annuity formula
![FV=P[((1+i)^n-1)/(i) ]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/c1km6xdnzppbq2d8dkmziozdbec5whz7dt.png)
where
FV is future value
now, we can plug values
![FV=75[((1+0.04)^(36)-1)/(0.04) ]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/h27leelhvu9kozv8zvmrsf070t1b9hv445.png)
we get

The money after 3 years is $5819.8735