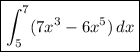No issues with convergence here. If you actually expand the summand you can use the well-known Faulhaber formulas to compute the sum.
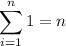
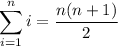
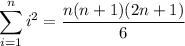

and so on. You would end up with
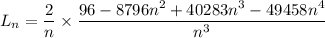
As
 , we have
, we have
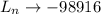 .
.
Now, when
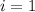 , the first term of the sum is
, the first term of the sum is

so the left endpoint of the first subinterval is
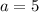 .
.
At the other end, when
 , the last term of the sum is
, the last term of the sum is
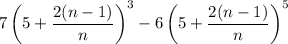
which converges to

so the left endpoint of the last subinterval converges to
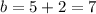 .
.
From here it's quite clear that
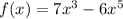 . So, the Riemann sum converges to the definite integral
. So, the Riemann sum converges to the definite integral