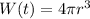Answer with explanation:
Let V be the volume of the tank.
Water is being poured into the spherical tank at a constant rate.
Let r, be the radius of spherical tank.
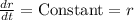
As, radius is constant. --------------------------------(1)
Volume of tank will increase with time.
So,⇒ Height of sphere=Radius of sphere
Volume of Spherical tank
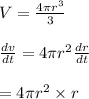
-----------------[using (1)]
So,