Answer:
k= 65 and 25
Explanation:
(a)
If the sequence is arithmetic then the common difference d between terms is equal
d = 5 - k = k - 125 ( subtract k from both sides )
5 - 2k = - 125 ( subtract 5 from both sides )
- 2k = - 130 ( divide both sides by - 2 )
k = 65
(b)
If the sequence is geometric then the common ratio r between terms is equal
r =
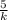 =
=
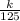 ( cross- multiply )
( cross- multiply )
k² = 625 ( take the square root of both sides )
k = ±
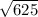 = ± 25
= ± 25
hence k = 25 ← positive value