Question

The boys mixed 10 gallons of the 20% pure lemon juice mix and 10 gallons of the 70% pure lemon juice mix, because they wanted 20 gallons of lemonade at a 40% lemon juice mixture. They thought that because 40% was almost halfway between 20% and 70%, they should just mix equal parts of both, but the lemonade turned out too tart. How much of each should they have used to get a final mixture of 20 gallons at 40% lemon juice? Write your answer in complete sentences. Show all work.

if you can help please show the work and have at least 1-2 paragraphs.

Answer 1

The correct quantities are 8 gallons of 70% and 12 gallons of 20%.

Explanation:

Boys have to prepare 20 gallons of 40% mixture using 20% and 70% mixtures.

Therefore, lemon in final mixture = 40% of 20 gallons = 8 gallons.

Let number of gallons taken from 20% mixture be x

So, Lemon contribution by this mixture = 20% of x gallons.

`=(x)/(5)\thinspace gallons.`

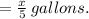
Now, since the mixture has to be 20 gallons, we have to take (20-x) gallons of 70% mixture.

Lemon contribution by this mixture =70% of (20-x)

`=14-((7\cdot x)/(10))\thinspace gallons`

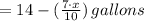
But, these both mixture should be added to get the final mixture :

`\implies(x)/(5) +14-((7\cdot x)/(10)) =8\\\\\implies (2-7)\cdot (x)/(10) = -6\\\\\implies x = 6* (10)/(5)\\\\\implies x = 12\thinspace gallons.`

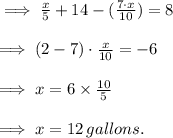
Thus, 20% mixture = 12 gallons and 70% mixture = 8 gallons