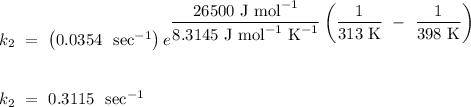Question

The rate constant, k, for a reaction is 0.0354 sec1 at 40°C. Calculate the rate constant for the

same reaction at 125°C if the activation energy is 26.5 kJ/mol.

Answer 1

The rate constant of the reaction at 125˚ is
`0.3115 \ \text{sec}^(-1)`.

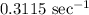Step-by-step explanation:

The Arrhenius equation is a simple equation that describes the dependent relationship between temperature and the rate constant of a chemical reaction. The Arrhenius equation is written mathematically as

`k \ = \ Ae^{\displaystyle(-E_(a))/(RT)}`

`\ln k \ = \ \ln A \ - \ \displaystyle(E_(a))/(RT)`

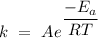
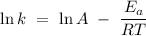
where
`k` is the rate constant,
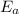`E_(a)` represents the activation energy of the chemical reaction,
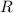`R` is the gas constant,
`T` is the temperature, and
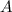`A` is the frequency factor.

The frequency factor,
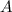`A`, is a constant that is derived experimentally and numerically that describes the frequency of molecular collisions and their orientation which varies slightly with temperature but this can be assumed to be constant across a small range of temperatures.

Consider that the rate constant be
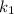`k_(1)` at an initial temperature
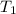`T_(1)` and the rate constant
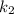`k_(2)` at a final temperature
`T_(2)`, thus

`\ln k_(2) \ - \ \ln k_(1) = \ \ln A \ - \ \displaystyle(E_(a))/(RT_(2)) \ - \ \left(\ln A \ - \ \displaystyle(E_(a))/(RT_(1))\right) \\ \\ \\ \rule{0.62cm}{0cm} \ln \left(\displaystyle(k_(2))/(k_(1))\right) \ = \ \displaystyle(E_(a))/(R)\left(\displaystyle(1)/(T_(1)) \ - \ \displaystyle(1)/(T_(2)) \right)`

`\rule{1.62cm}{0cm} \displaystyle(k_(2))/(k_(1)) \ = \ e^{\displaystyle(E_(a))/(R)\left(\displaystyle(1)/(T_(1)) \ - \ \displaystyle(1)/(T_(2)) \right)} \\ \\ \\ \rule{1.62cm}{0cm} k_(2) \ = \ k_(1)e^{\displaystyle(E_(a))/(R)\left(\displaystyle(1)/(T_(1)) \ - \ \displaystyle(1)/(T_(2)) \right)}`

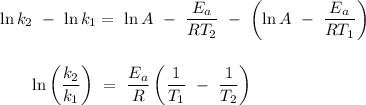
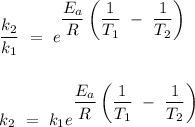
Given that
`E_(a) \ = \ 26.5 \ \ \text{kJ/mol}`,
`R \ = \ 8.3145 \ \ \text{J mol}^(-1) \ \text{K}^(-1)`,
`T_(1) \ = \ \left(40 \ + \ 273\right) \ K`,
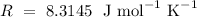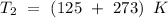`T_(2) \ = \ \left(125 \ + \ 273\right) \ K`, and
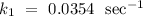`k_(1) \ = \ 0.0354 \ \ \text{sec}^(-1)`, therefore,

`k_(2) \ = \ \left(0.0354 \ \ \text{sec}^(-1)\right)e^{\displaystyle\frac{26500 \ \text{J mol}^(-1)}{8.3145 \ \text{J mol}^(-1) \ \text{K}^(-1)}\left(\displaystyle\frac{1}{313 \ \text{K}} \ - \ \displaystyle\frac{1}{398 \ \text{K}} \right)} \\ \\ \\ k_(2) \ = \ 0.3115 \ \ \text{sec}^(-1)`