Answer:
Accelerated motion is represented by a CURVED line on a nonlinear distance-time graph.
Step-by-step explanation:
For uniform acceleration we know that
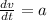
now integrating both sides we will have
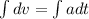
now after integration we will have
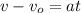
now we have
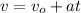
again we know that

again by integration both sides

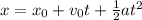
now since this equation is a quadratic equation so here distance time graph must be a curved graph