Answer:
Probability that 2 jars drawn at random will contain less jam is 0.00886524822
Explanation:
A jam manufacturer knows that in a box of 48 jars,5 will contain less jam than shown on the label. Now we have to find the probability that 2 jars drawn at random will contain less jam.
Given 5 jars contain less jam as shown on label. So 2 jars drawn at random from these 5 jars therefore number of ways or favourable outcome will be
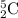
Total no. of outcomes=
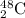
P(2 jars with less jam)=
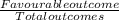
=
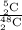
=
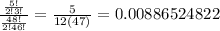
Hence, probability that 2 jars drawn at random will contain less jam is 0.00886524822