Question

HELP! Please!

Louise is building a guitar-like instrument. It has small metal bars, called frets, positioned across its neck so that it can produce notes of a specific scale on each string. The distance a fret should be placed from the bridge is related to the string’s root note length by the function d(n) = r ( 2^-n/12 ), where r is the length of the root note string and n is the number of notes higher than the string’s root note. Louise wants to know where to place frets to produce notes on a 50-cm string.

1. Find the distance from the bridge for a fret that produces a note exactly one octave (12 notes) higher than the root note .
a . Substitute values for r and n in the given function .
b . How far from the bridge should the fret be placed?
c . What fraction of the string length is the distance of this fret from the bridge?

Answer 1

Absolutely, let's go through these steps one by one and answer Louise's questions.

First, let's recall the given function for the distance a fret should be placed from the bridge in order to produce a note that is n notes higher than the string's root note:

\[ d(n) = r \cdot 2^{-\frac{n}{12}} \]

where \( r \) is the length of the root note string (in this case, 50 cm) and \( n \) is the number of notes higher than the string’s root note.

Now, Louise wants to place a fret that produces a note one octave higher than the root note. An octave is made up of 12 notes, which means we will use \( n = 12 \).

### Step 1 (a): Substitute values for \( r \) and \( n \) in the given function

We are given:

\( r = 50 \, \text{cm} \)
\( n = 12 \, \text{notes} \)

Substituting these values into the function, we have:

\[ d(12) = 50 \cdot 2^{-\frac{12}{12}} \]

### Step 1 (b): Calculate the distance from the bridge

Before we proceed with the calculation, let's simplify the exponent first:

\( 2^{-\frac{12}{12}} = 2^{-1} = \frac{1}{2} \)

Now, calculate the distance with the simplified exponent:

\[ d(12) = 50 \cdot \frac{1}{2} \]
\[ d(12) = 25 \, \text{cm} \]

This means that the fret should be placed 25 cm from the bridge.

### Step 1 (c): Calculate the fraction of the string length this distance represents

We found that the distance from the bridge is 25 cm, and we know that the total length of the string is 50 cm. To find the fraction, we divide the distance by the total length:

\[ \frac{d(12)}{r} = \frac{25 \, \text{cm}}{50 \, \text{cm}} \]

Simplify this fraction:

\[ \frac{d(12)}{r} = \frac{1}{2} \]

So, the distance of this fret from the bridge is \( \frac{1}{2} \) or 50% of the string's total length. This makes sense because an octave corresponds to a halving (or doubling if going the opposite way) of the string's length in a guitar or similar stringed instrument.

Answer 2

a.
`d(n)=50 * 2^{(-12)/(12) }`

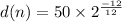
b. The fret should be placed 25 cm far from the bridge.

c. So, the fraction of string at which the fret is placed is
`(1)/(2)`.

Explanation:

We are given,

The function representing the distance of a fret from the bridge by
`d(n)=r * 2^{(-n)/(12) }`,

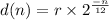where r = length of the root note string and n = number of notes higher than root note.

Now, Louis want to produce notes on a 50 com string. This gives r = 50.

Thus,
`d(n)=50 * 2^{(-n)/(12) }`.

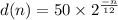1. It is required to produce notes which are 1 octave ( 12 notes ) higher than the root note. This gives that n = 12.

So, we get, r = 50 and n = 12 which gives us the function as,

a.
`d(n)=50 * 2^{(-12)/(12) }`

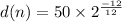
i.e.
`d(n)=50 * 2^(-1)`

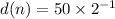
i.e.
`d(n)=(50)/(2)}`

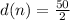
i.e.
`d(n)=25}`

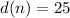
b. Thus, the fret should be placed 25 cm far from the bridge.

Now, as the fret is placed 25 cm far on the string having length 50 cm.

c. So, the fraction of string at which the fret is placed is
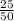`(25)/(50)` i.e.
`(1)/(2)`.