Answer:
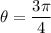
Explanation:
The formula for length of intercepted arc is
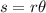
where, r is the radius of the circle and θ in the central angle in radian.
Radius of the circle = 48 millimeters
Length of intercepted arc = 36π millimeters
Substitute the given values in the above formula.
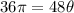
Divide both sides by 48.
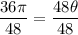
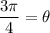
Therefore, the measure of central angle is
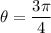 .
.