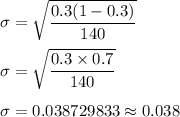Answer: 0.038
Explanation:
We know that for a sample size of N and a proportion of p , the standard deviation of the normal curve that can be used to approximate the binomial probability histogram is given by :-
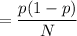
Given: p= 03.3
N= 140
Then , the standard deviation of the normal curve will be :-