Question

On the packaging for a triangular sail, the edge measurements for the sail are listed as

7 ft × 15 ft × 17 ft. Without unfurling the sail, you want to determine if the sail forms a right triangle, an acute triangle, or an obtuse triangle.

Answer 1

The sail forms an obtuse angle.

Explanation:

We will use Pythagorean theorem to classify triangle, which states:

• If the sum of squares of two shorter sides is smaller than the square of longest side than triangle is an obtuse triangle.

• If the sum of squares of two shorter sides is greater than the square of longest side than triangle is an acute triangle.

• If the sum of squares of two shorter sides is equal to the square of longest side than triangle is a right triangle.

Let us check our given sides using Pythagorean theorem.

`17^2?7^2+15^2`

`289?49+225`

`289>274`

We can see that the square of longest side is 289, which is greater than 274, therefore, the sail forms an obtuse angle.

Answer 2

Answer: Obtuse triangle.

Explanation:

1. By definition, you have:

- If the square of the length of the longest side is greater than the sum of the squares of the lengths of the other two sides, then the triangle is classified as "obtuse".

- If the square of the length of the longest side is less than the sum of the squares of the lengths of the other two sides, then the triangle is classified as "acute".

- If the square of the length of the longest side and the lenght of the other two sides are equal, then it is a right triangle.

2. Based on this information, you have:

`(17ft)^(2)=289ft^(2)\\(15ft)^(2)+(7ft)^(2)=274ft^(2)`

`289ft^(2)>274ft^(2)`

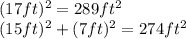
3. The longest side is greater than the other two sides. therefore, it is an obtuse triangle.