Question

If the rate of a reaction increases by a factor of 64 when the concentration of reactant increases by a factor of 4, what is the order of the reaction with respect to this reactant?

Answer 1

The reaction is of order three with respect to the reactant.

Step-by-step explanation

The rate of a reaction of order n about a certain reactant is proportion to the concentration of that reactant raised to the n-th power. This is true only if concentrations of any other reactants stay constant in the whole process.

In other words, Rate = constant × [Reactant]ⁿ, Rate ∝ [Reactant]ⁿ. (The symbol "∝" reads "proportional to".)

In this question,

[4 × Reactant]ⁿ ÷ [Reactant]ⁿ = 64.

In other words, 4ⁿ = 64, where n is the order of the reaction with respect to this reactant.

It might take some guesswork to find the value of n. Alternatively, n can be solved directly with a calculator using logarithms. Taking natural log of both sides:

`ln(4^n) = ln(64)\\n\; ln(4) = ln(64)\\n = (ln(64))/(ln(4)) = 3`.

Evaluating
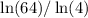`\ln(64) / \ln(4)` on Google or on a calculator with support for ln (the natural log) will give the value of n- no guesswork required.

n = 3. Therefore, the reaction is of order three with respect to this reactant.