Question

Jason determined that some teens like to eat vanilla ice cream, some like to eat chocolate ice cream, and others don't like to eat chocolate or vanilla. He calculated the probabilities and created the Venn diagram below: a venn diagram showing two categories, vanilla and chocolate. In the vanilla only circle is 0.4, in the chocolate only circle is 0.3, in the intersection is 0.2, outside the circles is 0.1 What is the probability that a teen eats vanilla ice cream, given that he/she eats chocolate ice cream?

Answer 1

20%

Explanation:

because the venn diagram is showing about 100% of his statistics. 0.1 is the people that don't like either. 0.2 is the people who like both. 0.3 is the chocolate only and 0.4 is the vanilla only. if we add those up we get 1. so 0.2 is 20% of 1.

Answer 2

Hence, the probability that a teen eats vanilla ice cream, given that he/she eats chocolate ice cream is:

2/5

Explanation:

Let A denote the event that teen eats Vanilla ice-cream.

B denote the event that teen eats Chocolate ice-cream.

and A∩B denote the event that teen eats both chocolate and vanilla ice-cream.

Now , let P be the probability of an event.

We are asked to find:

P(A|B)

We know that the probability i.e. P(A|B) is given by:

`P(A|B)=(P(A\bigcap B))/(P(B))`

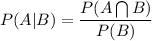
We are given:

Let x be the total number of people who are surveyed.

We have:

A=0.6 x.

B=0.5 x

A∩B=0.2 x

Hence,

`P(A∩B)=(0.2x)/(x)=0.2`

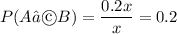
and,

`P(B)=(0.5x)/(x)=0.5`

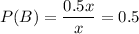
( Since, 0.2+0.3=0.5)

Hence,

P(A|B)=0.2/0.5

i.e.

P(A|B)=2/5