For this case we have that by definition, the sum of the internal angles of a triangle is 180 degrees.
Then, according to the figure djunta we have:
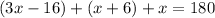
Grouping similar terms:
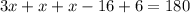
We clear the value of x:
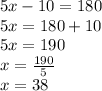
Then the angle M is given by:

Substituting the value of x we have:

Thus, the angle M is 98 degrees.
Answer:
98 degrees