Question

Ten granola bars and twelve bottles of water cost $23. Five granola bars and four bottles of water cost $10. How much do one granola bar and one bottle of water cost?

Answer 1

$2.15

Explanation:

Answer 2

$2.15

Explanation:

Given :

The cost of Ten granola bars and twelve bottles of water is $23.

The cost of Five granola bars and four bottles of water is $10.

To Find : The cost of one granola bar and one bottle of water

Solution :

Let x be the cost of one granola bar.

Let y be the cost of one water bottle.

So, cost of 10 granola bars = $10 x

Cost of 5 granola bars = $5 x

Cost of twelve bottles of water = $12 y

Cost of four bottles of water = $4 y

Now we know that the cost of Ten granola bars and twelve bottles of water is $23.

So, the equation becomes:

⇒
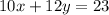`10x+12y=23` ---(a)

We also know that cost of Five granola bars and four bottles of water is $10.

So, the equation becomes:

⇒
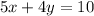`5x+4y=10` ---(b)

Now we need to solve (a) and (b) to find value of x and y

So, we will use substitution method

We will substitute value of x from (a) in (b)

⇒
`5((23-12y)/(10) )+4y=10`

⇒
`(23-12y)/(2) +4y=10`

⇒
`11.5-6y+4y=10`

⇒
`11.5-2y=10`

⇒
`11.5-10=2y`

⇒
`1.5=2y`

⇒
`(1.5)/(2) =y`

⇒
`0.75 = y`

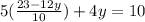
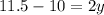
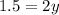
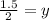
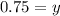
So, cost of one water bottle 'y' is $0.75

Now we are supposed to find the value of x . So, put value of y in equation (a)

⇒
`10x+12(0.75)=23`

⇒
`10x+9=23`

⇒
`10x=23-9`

⇒
`10x= 14`

⇒
`x= (14)/(10)`

⇒
`x= 1.4`

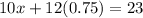
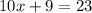
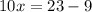
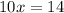
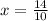
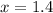
So, cost of one granola bar 'x' is $1.4

So, combine cost of 1 granola bar and one water bottle is $1.4+ $0.75 = $2.15