Answer:
y=3 and m=
 .
.
Explanation:
We are given two lines y=6x+15 and y=mx-4.
x-coordinate of the intersection point is x=-2.
In order to find the y-coordinate of intersection point, we need to plug x=-2 in first equation.
Plugging x=-2 in y=6x+15, we get
y=6(-2)+15
y=-12+15
y=3.
Therefore, y-coordinate of the intersection point is y=3.
Now, in order to find the value of m, we need to plug x and y-coordinate values in second equation and solve for m.
Plugging x=-2 and y=3 in second equation y=mx-4, we get
3=m(-2)-4
3 = -2m -4
Adding 4 on both sides, we get
3+4=-2m-4+4
7=-2m.
Dividing both sides by -2, we get.
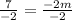

Therefore, m=
 .
.