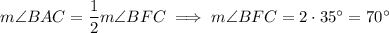The inscribed angle theorem says that the angle formed by two intersecting chords of a circle (the angle A between the chords AC and AB in this case) has half the measure of the central angle subtended by the arc containing those chords (arc CB in this case). So