Question

In parallelogram ABCD , diagonals AC⎯⎯⎯⎯⎯ and BD⎯⎯⎯⎯⎯ intersect at point E, AE=x2−16 , and CE=6x .

What is AC ?

Answer 1

96

Explanation:

just took the test

Answer 2

AC=96 units.

Explanation:

We are given a parallelogram ABCD with diagonals AC and BD intersect at point E.

`AE=x^2-16.` , and CE=6x .

Note: The diagonals of a parallelogram intersects at mid-point.

Therefore, AE = EC.

Plugging expressions for AE and EC, we get

`x^2-16=6x.`

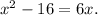
Subtracting 6x from both sides, we get

`x^2-16-6x=6x-6x`

`x^2-6x-16=0`

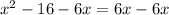
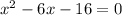
Factoriong quadratic by product sum rule.

We need to find the factors of -16 that add upto -6.

-16 has factors -8 and +2 that add upto -6.

Therefore, factor of
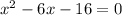`x^2-6x-16=0` quadratic is (x-8)(x+2)=0

Setting each factor equal to 0 and solve for x.

x-8=0 => x=8

x+2=0 => x=-2.

We can't take x=-2 as it's a negative number.

Therefore, plugging x=8 in EC =6x, we get

EC = 6(8) = 48.

AC = AE + EC = 48+48 =96 units.