Answer:
11) Here given Function,
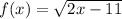
And,
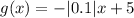
For f(x) = g(x)
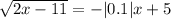
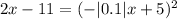
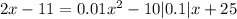
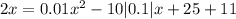
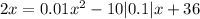
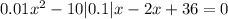
When we solve this equation,
We found,
x = 12.5227 ≈ 12.53
Thus, the required solution is, x = 12.53
12) Here the height of rocket A in x second,
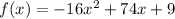
And, The height gain by the rocket B in x seconds,
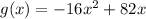
If at x seconds both A and B gain the same height,
That is, f(x) = g(x)
⇒
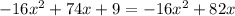
⇒

⇒
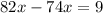
⇒
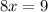
⇒ x = 1.125 ≈ 1.13
Thus, the required solution is x = 1.13 seconds (approx)