Question

the diffrence of two numbers,a and b,is 21,the diffrence of five times a and two times b is 18 what are the values of a and b

Answer 1

Values of a and b are;

a = -8 and b = -29

Explanation:

As per the statement:

Difference of two numbers, a and b is 21

i.e, a -b = 21 .......[1]

Also, it is given that the difference of five times a and two times b is 18.

"five times a" means 5a

"two times b" means 2b

then;

5a - 2b = 18 ......[2]

We can write equation [1] as;

a = b + 21 .....[3]

Substitute the value of a in equation [2] we have;

`5(b+21)-2b =18`

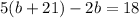
Using distributive property;
`a\cdot(b+c) = a\cdot b + a\cdot c`

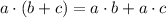
5b + 105 - 2b = 18

combine like terms we get;

3b = 18 -105

Simplify:

3b = -87

Divide by 3 on both sides we get;

b = -29

Substitute the value of b in equation [3] to solve for a;

a = -29 + 21 = -8

Therefore, the values of a and b is, -8 and -29

Answer 2

a=-8

b=-29

Explanation:

Let's assume

first number is a

second number is b

the difference of two numbers,a and b,is 21

so, we get

`a-b=21`

we can solve for 'a'

`a=b+21`

the difference of five times a and two times b is 18

so, we get

`5a-2b=18`

now, we can plug 'a'

`5(b+21)-2b=18`

now, we can solve for b

`5b+105-2b=18`

`3b+105=18`

`3b=-87`

`b=-29`

now, we can find 'a'

`a=-29+21`

`a=-8`