Answer:
As per the given statement: A straight road rises at an inclination of 0.3 radian from horizontal.
⇒Inclination
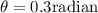 .
.
The slope is given by:
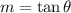
Substitute the given value we have;
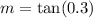
m = 0.30933624961
Let Δx represents the change in horizontal distance and Δy represents the change in vertical distance.
Slope is also defined as the change in vertical distance to the change in horizontal distance.
i.,e
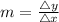 .....[1]
.....[1]
It is given that change in elevation over a one mile section of the road
⇒Δx = 1
Substitute the value of Δx = 1 and m = 0.30933624961 in [1] we have;
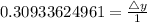
or
 mile.
mile.
Therefor, the slope is, 0.30933624961 and change in elevation over a one mile section of the road is, 0.30933624961 mile