Answer:
The coordinates of point P are (-1, -1)
Explanation:
The coordinates of the partition point (x, y) of a line segment whose endpoints are (x1, y1) and (x2, y2) at ratio m: n are
- x =
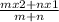
- y =
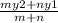
∵ P partitions segment HS in a ratio 2: 3
∴ P = (x, y)
∴ m = 2 and n = 3
∵ H = (5, -9) and S = (-10, 11)
∴ x1 = 5 and x2 = -10
∴ y1 = -9 and y2 = 11
→ Substitute them in the rule above to find x and y
∵ x =
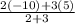 =
=
 =
=
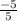
∴ x = -1
∵ y =
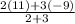 =
=
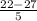 =
=
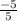
∴ y = -1
∴ The coordinates of point P are (-1, -1).