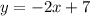Answer:
y = -2x+7
Explanation:
equation of line formula:
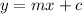
m = slope, c = y-intercept
1) find the slope using the given coordinates
slope formula:
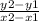
i) (3,1)
x1=3
y1=1
ii) (7,-7)
x2=7
y2=-7
substitute the values into the formula


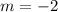
so now half of the equation is complete as we have the the value of m. substitute the value of m into the slope formula.
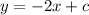
2) find the value of c
to find the value of c, we must substitute either coordinate into the half equation. so, i choose the coordinate (3,1). if you substitute the coordinate (7,-7) , you will get the same value of c.
(3,1)
x = 3
y = 1
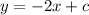

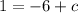
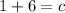
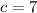
substitute the value of c into the half equation and that is your straight line equation